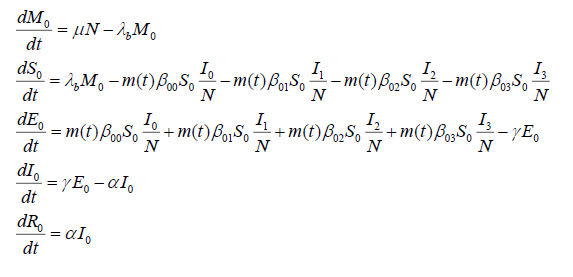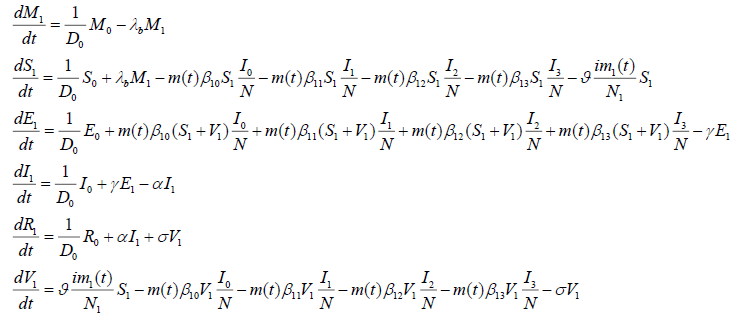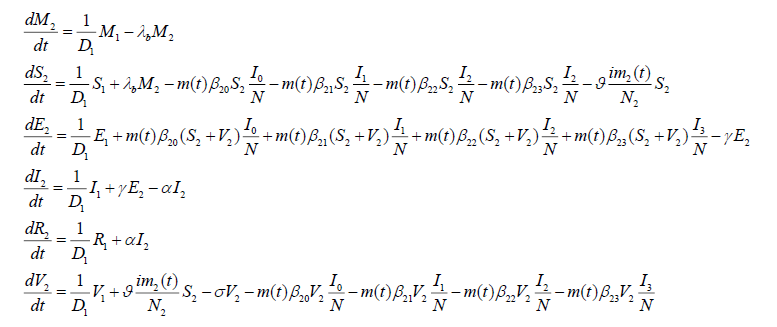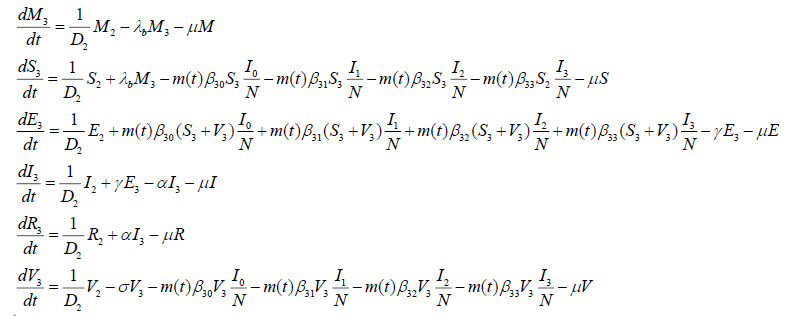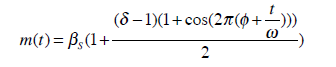Notice: This Wiki is now read only and edits are no longer possible. Please see: https://gitlab.eclipse.org/eclipsefdn/helpdesk/-/wikis/Wiki-shutdown-plan for the plan.
Measles Transmission Model
Background
The immunization is decreasing.
Model
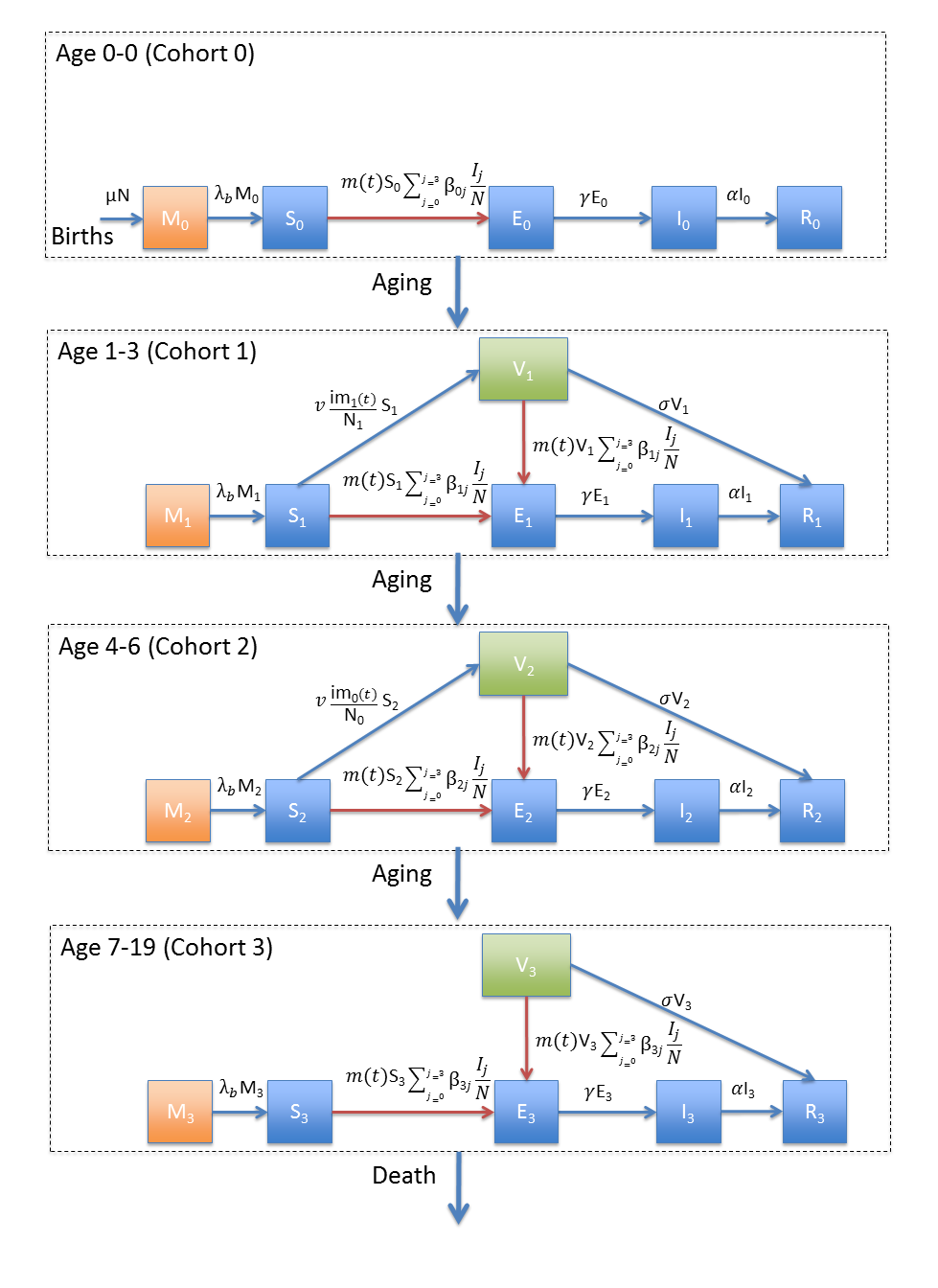
An MSEIRV model is developed to study the spread of measles and the effect of vaccination. M,S,E, I,R and V represent the maternal immunity, susceptible, exposed, infected, recovered and vaccinated populations respectively. N is the total population size which is the summation of all children and adolescents in all these states at a given time period (N=M+S+E+I+R+V). Figure 1 below shows the flow of the model with the rate parameters. This model can be applicable to any MSEIR disease model where vaccinations are available.
Fig. 1 Compartment Model of Measles Transmission in STEM
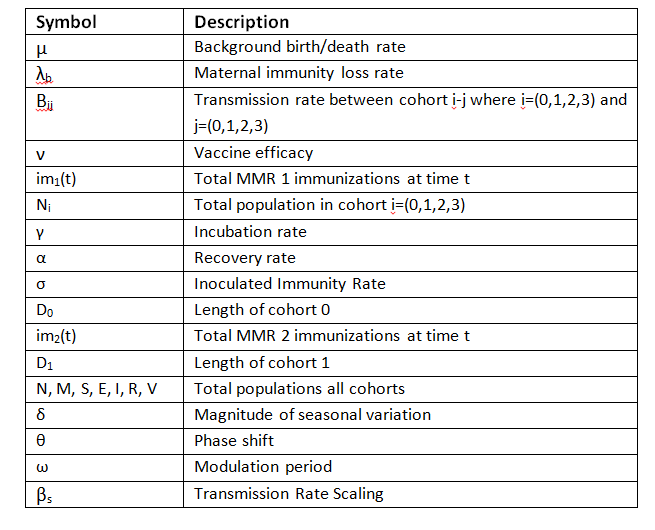
For this example, there are 4 cohorts: Ages 0-0 (Cohort 0), Ages 1-3 (Cohort 1), Ages 4-6 (Cohort 2) and Ages 7-19 (Cohort 3). Age of vaccination changes for different countries. In general, the first dose of MMR is registered around age 1 and the second dose is registered around age 5. Also, in addition to preschool children, measles cases are common in the adolescents. Thus, these three cohorts are included in the model. One might only use one cohort depending on the aim of the model and the data available. The maternal immunity would disappear by the age of 3. Once a model is built for a cohort in STEM, it replicates the structure for the other cohorts. The description of the parameters are given in Table 1 below. While most of the parameters can be find from previous studies, transmission scaling parameter is found by fitting the model to the historical data.
Table 1 Table of Parameters
Below are the differential equations that explain the rate change in the system. Sets 1-4 represent the rate change in the cohorts 0-3. Last equation expresses how to include effect of seasonality in transmission of measles.
Fig. 2 Ordinary Differential Equations of Measles Transmission Model